Answer:
There are 49,000,00,000 possible numbers.
49,000,00,000 números diferentes posibles.
Explanation:
If we have n trials, each with m possible outcomes, the total number of possible outcomes is:
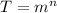
In this question:
The first two trials(numbers), 3 digits are not possible in each. So 7 are possible.
The last eight numbers, all 10 digits are possible.
Then
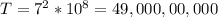
There are 49,000,00,000 possible numbers.
49,000,00,000 números diferentes posibles.