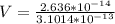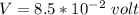Answer:
The potential difference is
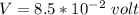
Step-by-step explanation:
From the question we are told that
The diameter of the of both plates is
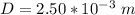
The distance of separation is

The potential difference is
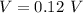
The new charge on the plate is
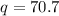 % of
% of
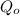
Where
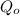 is the original charge on the capacitor
is the original charge on the capacitor
Generally the charge on the capacitor is mathematically represented as
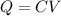
Where C is the capacitance of the capacitor which is mathematically represented as
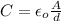
Where
 is the permittivity of free space which is a constant with value
is the permittivity of free space which is a constant with value
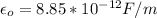
A is the area which is mathematically represented as

substituting values
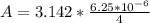

Now
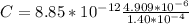
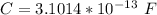
So


Generally

Here

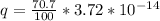
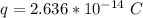
Making V the subject in the above formula
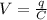
Substituting values