Question

A block 0.1 m square, with 5 kg mass, slides down a smooth incline, 300 below the horizontal, on a film of SAE 30 oil at 200C that is 0.20 mm thick. If the block is released from rest at t = 0, what is its initial acceleration? Derive an expression for the speed of the block as a function of time. Plot the curve for V(t). Find the speed after 0.1 s. If we want the block to instead reach a speed of 0.3 m/s at this time, find the viscosity µ of the oil we would have to use.

Answer 1

a. acceleration (a) = 4.9 m/s²

b.
`V(t) = (mgdsin \theta)/(uA)(1-(uA)/(e^(md)) t)`

c. see the attached diagram below

d V = 0.048 m/s

e. The viscosity u = 0.27 Ns/m²

Step-by-step explanation:

Given that :

m = 5 kg

A = ( 0.1 m )²

d = 0.20 mm

θ = 30 °

By applying Newton's second Law ; we have

F = mgsinθ -
`F_f` (since no friction ; then

ma = mg sinθ

a = gsin θ

a = 9.8 × sin 30

a = 4.9 m/s²

b) By applying Newton's second law at any instant .

ma = mgsin θ -
`F_f`

and
`F_f` = T×A =
`u (dv)/(dy)A` -
`u (v)/(d)A`

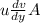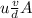
Also; ma =
`m (dv)/(dt)`

∴ ma = mg sin θ -
`(u A)/(d)V`

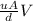
separating the variables ; we have

`(dv)/(gsin \theta - (uA)/(md)V) = {dt}`

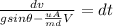
By integrating using limits ; we have:

`(m.d)/(uA) \lim(1-(uA)/(mgdsin \theta)V)= t}`

∴
`V(t) = (mgdsin \theta)/(uA)(1-(uA)/(e^(md)) t)`

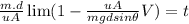
c) The curve for V(t) plot is attached in the diagram below.

d)

To find the speed after 1 sec; we have:

at t = 1.0 sec;

V =
`(5*9.81*0.0002sin (30))/(0.4*(0.1)^2)(1-e^{-(0.4*0.01)/(5*0.002)*0.1})`

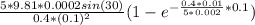
V = 0.048 m/s

e) In order to determine the viscosity for which V(0.1) = 0.3 m/s ; we have the following.

∴
`v(t= 0.3) = (mgd sin \theta )/(uA)(1-e^{(uA)/(md)(t=0.1)})`

`0.3 = (5*9.81*sin 30*0.0002)/(u(0.1)^2)(1-e^{(u*0.01)/(5*0.0002)(0.1)})`

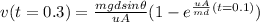
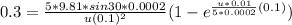
The viscosity u = 0.27 Ns/m²