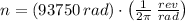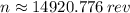Answer:
a)
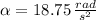 , b)
, b)
Step-by-step explanation:
a) The final angular speed is:
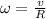
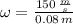
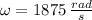
The angular acceleration experimented by the centrifuge is determined by means of the following kinematic expression:
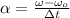
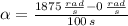
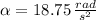
b) The change in angular position is:
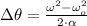
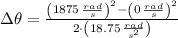
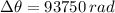
Lastly, the total amount of revolutions made by the centrifuge is: