Answer:
5.79*10⁻⁹ m is the uncertainty in the position.
Step-by-step explanation:
Heisenberg's uncertainty principle assumes that it is not possible to know exactly all the data regarding the behavior of particles. In other words, at the subatomic level, it is impossible to know at the same moment where a particle is, how it moves and what its speed is.
So, Heisenberg's Uncertainty Principle gives a relationship between the standard deviation of an object's position and its momentum.
Δp*Δx= h/(4π)
where
- Δp the standard deviation of the object's momentum,
- Δx the standard deviation of the object's position,
- h=6.63*10⁻³⁴ J.s is the Planck's constant.
By definition, the momentum of the electron equals the product of its mass and velocity. So, being the mass constant, you can said:
Δp= m*Δv
Replacing in the expresion of the Heisenberg's Uncertainty Principle:
m*Δv*Δx= h/(4π)
Then you know:
- m=9.11*10⁻³¹ kg
- Δv=0.01*10⁶ m/s
- h=6.63*10⁻³⁴ J.s= 6.63*10⁻³⁴ (N*m)*s=6.63*10⁻³⁴ [(kg*m*s⁻²)*m]*s= 6.63*10⁻³⁴ kg*m²*s⁻¹
Replacing:
*Δx=6.63*10⁻³⁴ kg*m²*s⁻¹/(4π)
Taking π=3.14 and solving:
Δx
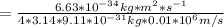
Δx=5.79*10⁻⁹ m
5.79*10⁻⁹ m is the uncertainty in the position.