Question

A soda manufacturer knows that sales of soda increase during the summer. To make sure that they get a large portion of the sales, they decide to offer a contest throughout the spring. The contest is pretty simple: when you open your bottle of soda, if it says, “You win!” under the cap, you get another bottle of their soda for free. The chance of winning a free soda is 1 in 12. Lucky Lucy bought five sodas and won a free one with four of the caps. What’s the probability of that happening? Theoretically, how many sodas would you have to buy before having a greater than 50% chance of winning a free soda?

Answer 1

Explanation:

Hello!

The variable of interest is

X: Number of winning caps out of 5 bottles.

Checking the binomial criteria:

- Number of trials is fixed n=5 bottles of soda

- Only two possible outcomes per trial: "success": winning cap; "failure": regular cap.

- The trial are independent.

- The probability of success is constant trough the experiment p= 1/12= 0.083

This variable has a binomial distribution with parameters n= 5 and p= 0.083

You have to calculate the probability of getting 4 out of 5 winning caps

P(X=4)

Using the following formula:

`P(X=4)= (n!)/((n-X)!X!) * (p)^(X) * (q)^(n-X)= (5!)/(1!4!) * (0.083)^(4) * (0.917)^(1)= 0.0002`

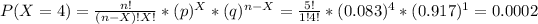
Or using the tables for accumulated probabilities:

P(X=4)= P(X≤4)-P(X≤3)= 0.9999 - 0.9997= 0.0002