Question

Erica's parents gave her $500 for her high school graduation. She put the money into a savings account that earned 7.5% annual interest. She left the money in the account for nine months before she withdrew it. About how many months would she have to leave the money in the account if she wants to reach her goal of saving $750? Give your answer in months.

Answer 1

She would need to leave the money in her account for 6 years and 8 months.

Explanation:

This is a simple interest problem.

The simple interest formula is given by:

`E = P*I*t`

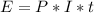
In which E are the earnings, P is the principal(the initial amount of money), I is the interest rate(yearly, as a decimal) and t is the time.

After t years, the total amount of money is:

`T = E + P`.

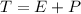In this question:

She put 500 into the saving account, so
`P = 500`

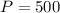
7.5% annual interest, so
`I = 0.075`

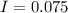
Saving $750. So we need T = 750.

Interest earned:

`T = E + P`

`750 = E + 500`

`E = 250`

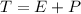
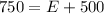
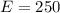
How long to earn $250 in interest, in years:

`E = P*I*t`

`250 = 500*0.075*t`

`t = (250)/(500*0.075)`

`t = 6.67`

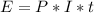
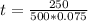
Converting to months:

6.67 years, that is, 6 years and (2/3) of an year.

An year has 12 months.

(2/3)*12 = 8.

So

She would need to leave the money in her account for 6 years and 8 months.