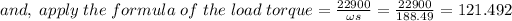Question

The power crossing the air gap of a 60 Hz, four-pole induction motor is 25 kW, and the power converted from electrical to mechanical form in the motor is23.4 kW. (a)What is the slip of the motor at this time? (b)What is the induced torque in this motor? (c)Assuming that the mechanical losses are 300 W at this slip, what is the load torque of this motor?

Answer 1

a) - 0.064.

b) - 1240.14.

c) - 121.492.

Step-by-step explanation:

Given,

Let the frequency to be f = 60 Hz

Let Pag = 25 kW and Pm = 23.4 kW.

a) - The motor slip during this moment is 0.064.

Apply the formula of the Pm.

`Pm=(1-slip)\;Pag`

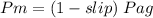
Then, put the value in the above equation.

`23.4=(1-slip)* 25`

`1-slip=(23.4)/(25)`

`slip=1-0.936=0.064`.

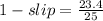
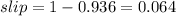b) - The induced torque within that motor is 124.14.

`Ns=(120f)/(p) =(120*60)/(4) =1800rpm`

`\omega s=188.49`

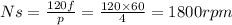
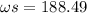
Then, we have to apply the formula of the induced torque.

`Induced\;Torque=(Pm)/(\omega s)=124.14`

c) - Let the mechanical losses to m = 300 W.

`Then,\;we\;have\;to\;find\;the\;out put\;power=23200-300=22900`

`and,\;apply\;the\;formula\;of\;the\;load\;torque =(22900)/(\omega s) =(22900)/(188.49)=121.492`