Question

Calculate the ratio of Htions to OH-ions at a pH = 6

Find the concentration of H+ ions to OH-ions listed in
Table B of your Student Guide. Then divide the H+
concentration by the OH concentration. Record this
calculated ratio in Table A of your Student Guide.
Compare your approximated and calculated ratios of H+
ions to OH-ions at a pH = 6. Are they the same? Why or
why not? Record your explanation in Table A.
What is the concentration of H+ ions at a pH = 6?
moll
What is the concentration of OH ions at a pH = 6?
mol/L
What is the ratio of H+ ions to OH ions at a pH = 6?
1

Answer 1

At a pH of 6, the concentration of H+ ions is 1 x 10^(-6) M and the concentration of OH- ions is 1 x 10^(-8) M. The ratio of H+ ions to OH- ions at a pH 6 is 100.

The concentration of H+ ions at a pH 6 is calculated using the formula: pH = -log[H+].

Rearranging the formula, we get [H+] = 10^(-pH).

Substitute the pH value of 6 into the equation: [H+] = 10^(-6) = 1 x 10^(-6) M.

Therefore, the concentration of H+ ions at a pH 6 is 1 x 10^(-6) M.

To find the concentration of OH- ions at a pH 6, we use the equation: [H+] x [OH-] = 1
`x 10^(-14) M^2.`

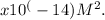
Since we know the concentration of H+ ions is 1 x 10^(-6) M, we can substitute this value into the equation: (1 x 10^(-6)) x [OH-] = 1 x 10^(-14).

Divide both sides of the equation by 1 x 10^(-6) to solve for [OH-]: [OH-] = 1 x 10^(-14) / 1 x 10^(-6) = 1 x 10^(-8) M.

Therefore, the concentration of OH- ions at a pH 6 is 1 x 10^(-8) M.

The ratio of H+ ions to OH- ions at a pH 6 can be calculated by dividing the concentration of H+ ions by the concentration of OH- ions: (1 x 10^(-6)) / (1 x 10^(-8)) = 100.

The ratio of H+ ions to OH- ions at a pH 6 is therefore 100.

Answer 2

1. 1×10^-6 mol/L

2. 1×10^-8 mol/L

3. 100

Step-by-step explanation:

Data obtained from the question include:

pH = 6

Please see attachment for explanation on how to arrive at the answer.