Answer:
-The equation for the following problem:

Explanation:
-The equation of a circle:
 (where
(where
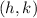 represents the center and
represents the center and
 represents the radius).
represents the radius).
-According to following circle on a graph, the center of the circle is
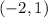 and to find the radius you need to use the center and the point on a circle, which I pick a coordinate
and to find the radius you need to use the center and the point on a circle, which I pick a coordinate
 , to solve the equation and get the radius:
, to solve the equation and get the radius:
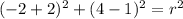
Solve:
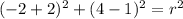
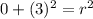

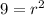
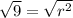
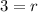
-So, after you found the radius, use the center and the radius to make the equation of a circle:
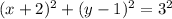
When putting the center and the radius on the equation of a circle, the radius needs to be simplified by the exponent and now you have found the answer for the following circle on a graph:

-There is one trick to get the center and the radius, look at the center of the circle if you see the coordinate that is in middle, then that would be the coordinate for the center, which is
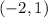 . To find the radius, count from the center, which is coordinate
. To find the radius, count from the center, which is coordinate
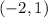 to a point that lands on a circle, which is
to a point that lands on a circle, which is
 . Since the distance is
. Since the distance is
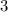 , simplify the
, simplify the
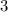 by the exponent
by the exponent
 , because the radius is
, because the radius is
 from the equation of a circle and you get the radius
from the equation of a circle and you get the radius
 .
.