Answer:
(0.3, -18.45).
Explanation:
We need to recur to the extreme value theorem, which states: "If a function is continuous on a closed interval, then that function has a maximum and a minimum inside that interval".
Basically, as the theorem states, if a dunction is continuous, then it has maxium or minium.
In this case, we have a quadratic function, which is a parabola. An important characteristic of parabolas is that they have a maximum or a minium, but they don't have both. When the quadratic term of the fuction is positive, then it has a minium at its vertex. When the quadratic term of the function is negative, then it has a maximum at its vertex.
So, the given function is
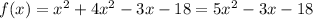 , where the quadratic term is positive, so the functions has a minimum at
, where the quadratic term is positive, so the functions has a minimum at
 , where
, where
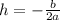 and
and
 , let's find that point
, let's find that point
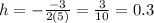
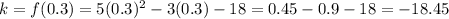
Therefore, the minium of the function is at (0.3, -18.45).