Question

You are driving on a hot day when your car overheats and stops running. The car overheats at 280°F and can be driven again at 230°F. When it is 80°F outside, the cooling rate of the car is r=0.0058​ . How long do you have to wait until you can continue driving? Use Newton's Law of Cooling to solve the problem. Round your answer to the nearest whole minute.

Answer 1

50 minutes

Explanation:

Given:

Temperature, T = 230°F

Overheat temp, To = 280°F

Ts = 80°F

Cooling rate, r = 0.0058

Since the car can be driven again at 230°F, and when it is 80°F outside, the cooling rate of the car is 0.0058, using Newton's law of cooling, we have:

`T = (T_o - T_s)​ e^-^r^t + T_s`

Substituting figures, we have:

`230 = (280 - 80)​ e^-^0^.^0^0^5^8^t + 80`

`230 = 200e^-^0^.^0^0^5^8^t + 80`

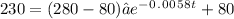
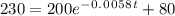
Solving further, let's subtract 80 from both sides, we now have:

`230 - 80= 200e^-^0^.^0^0^5^8^t + 80 - 80`

`150 = 200e^-^0^.^0^0^5^8^t`

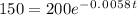
Divide both sides by 200:

`(150)/(200)= (200e^-^0^.^0^0^5^8^t)/(200)`

`= (150)/(200)=e^-^0^.^0^0^5^8^t`

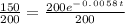
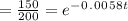
Let's take the natural log of both sides, we have:

`In 0.75 = In e^-^0^.^0^0^5^8^t`

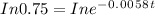
Using logarithm power rule, we have:

= - 0.2877 = - 0.0058t

To find t, let's now divide both sides by -0.0058

`(-0.2877)/(-0.0058) = (-0.0058t)/(-0.0058)`

50 = t

Therefore, you have to wait for 50 minutes before you can continue driving.