Answer:
Probability of having a sample mean of 114.6 or less is 0.8315.
Explanation:
We are given that worldwide organization of academics claims that the mean IQ score of its members is 112, with a standard deviation of 16.
A randomly selected group of 35 members of this organization is tested, and the results reveal that the mean IQ score in this sample is 114.6.
Let
 = sample mean
= sample mean
The z score probability distribution for sample mean is given by;
Z =
 ~ N(0,1)
~ N(0,1)
where,
 = population mean IQ score of its members = 112
= population mean IQ score of its members = 112
 = standard deviation = 16
= standard deviation = 16
n = sample of members = 35
Now, probability of having a sample mean of 114.6 or less is given by = P(

 114.6)
114.6)
P(

 114.6) = P(
114.6) = P(


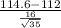 ) = P(Z
) = P(Z
 0.96)
0.96)
= 0.8315
Hence, the required probability is 0.8315.