Question

Which triangle would be most helpful in finding the distance between the points (-4,3) and (1, -2) on the coordinate

plane?

Answer 1

Right Triangle, the distance between these two coordinates is 5.83 in whatever unit you're measuring.

Explanation:

Generally when you're using triangles to find the distance between two graphed points, you're using a right triangle.

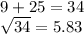`9 + 25 = 34\\√(34)=5.83`The reason behind that being you can draw lines on the x and y axis of these points and find where they connect. After doing so, you can directly connect the two points and create a right triangle.

To find the distance between the two points using a right triangle, we can use the formula for the hypotenuse:
`a^2 + b^2=c^2`

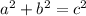
A and B is the distance between the point and where the lines you've drawn intersect. C is the resulting distance between the two points.

Plugging in A and B, which is 3 and 5, we can find C. 3 squared is 9 and 5 squared is 25.

`3^2 + 5^2 = 9 + 25`

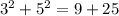
Now we add the two and find the square root.

`9+25=34\\√(34) =5.83`

Your answer is 5.83