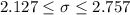Question

83 random samples were selected from a normally distributed population and were found to have a mean of 32.1 and a standard deviation of 2.4. Construct a 90% confidence interval for the population standard deviation.

Answer 1

`((82)(2.4)^2)/(104.139) \leq \sigma^2 \leq ((82)(2.4)^2)/(62.132)`

`4.525 \leq \sigma^2 \leq 7.602`

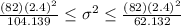
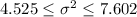
Now we just take square root on both sides of the interval and we got:

`2.127 \leq \sigma \leq 2.757`

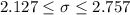
Explanation:

Information given

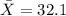`\bar X=32.1` represent the sample mean

`\mu` population mean (variable of interest)

s=2.4 represent the sample standard deviation

n=83 represent the sample size

Confidence interval

The confidence interval for the population variance is given by the following formula:

`((n-1)s^2)/(\chi^2_(\alpha/2)) \leq \sigma^2 \leq ((n-1)s^2)/(\chi^2_(1-\alpha/2))`

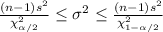
The degrees of freedom given by:

`df=n-1=8-1=7`

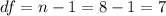
The confidence level is 0.90 or 90%, the value of
`\alpha=0.1` and
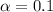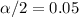`\alpha/2 =0.05`, and we can use excel, a calculator or a table to find the critical values.

The excel commands would be: "=CHISQ.INV(0.05,82)" "=CHISQ.INV(0.95,82)". so for this case the critical values are:

`\chi^2_(\alpha/2)=104.139`

`\chi^2_(1- \alpha/2)=62.132`

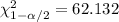
The confidence interval is given by:

`((82)(2.4)^2)/(104.139) \leq \sigma^2 \leq ((82)(2.4)^2)/(62.132)`

`4.525 \leq \sigma^2 \leq 7.602`

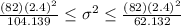
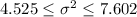
Now we just take square root on both sides of the interval and we got:

`2.127 \leq \sigma \leq 2.757`