Question

Correct answer.

Consider the two similar cylinders. The heights of both cylinders are given, along with the diameter of the smaller cylinder. What is the diameter
of the larger cylinder?
48 in
32 in
12 in
Figures not drawn to scale
А
28 inches
B
20 inches
18 inches

Answer 1

18 inches

Explanation:

Given: The heights of both cylinders are 48 inches and 32 inches. Diameter of the larger cylinder is 12 inches

To find: diameter of the larger cylinder

Solution:

Height of the larger cylinder (H) = 48 inches

Height of the smaller cylinder (h) = 32 inches

Diameter of the smaller cylinder (d) = 12 inches

Radius of the smaller cylinder (r) = d/2 =12/ 2= 6 inches

As two cylinders are similar, their heights and radii are proportional.

`(r)/(h)=(R)/(H)\\(6)/(32)=(R)/(48)\\R=(6)/(32)* 48=9\,\,inches`

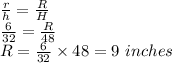
Diameter of the larger cylinder = 2R = 2(9) = 18 inches