Question

Item 25

25) Solve the problem.
An investment is worth $3733 in 1994. By 1997 it has grown to $5425. Let y be the value of
the investment in the vear x, where x 0 represents 1991. Write a linear equation that
models the value of the investment in the vear x.
y = -564x + 7117
1 x + 3733
564

Answer 1

`m =(y_2 -y_1)/(x_2-x_1)= (5425-3733)/(3-0)= 564`

Now we can use the value for 1994 and we can find the intercept like this:

`3733 = 564*0 +b`

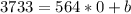
And solving for b we got:

`b = 3733 -0= 3733`

So then oir model would be given by:

`y= 564x +3733`

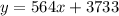
Option C

Explanation:

For this case we want to create a linear function for the the value of the investment in the vear x, where x =0 represents 1994.

We know that for 1994 (x= 0) the value is y = $3733 and for 1997 (x=3) the value of y = $5425

We want to find a model given by:

`y = mx +b`

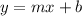
Where m is the slope and b the intercept. We can find the slope with this formula:

`m =(y_2 -y_1)/(x_2-x_1)= (5425-3733)/(3-0)= 564`

Now we can use the value for 1994 and we can find the intercept like this:

`3733 = 564*0 +b`

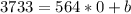
And solving for b we got:

`b = 3733 -0= 3733`

So then oir model would be given by:

`y= 564x +3733`

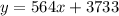
Option C