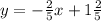Question

Question
Find an equation for the line that passes through the points (-4,3) and (6,-1)

Answer 1

`y = - (2)/(5) + 1 (2)/(5)`

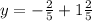
Explanation:

The equation of a line is usually written in the form of y=mx+c, where m is the gradient and c is the y-intercept

To find the gradient, use the gradient formula below.

`gradient = (y1 - y2)/(x1 - x2)`

`m = (3 - ( - 1))/( - 4 - 6) \\ m = (4)/( - 10) \\ m = - (2)/(5)`

`y = - (2)/(5) x + c`

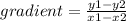
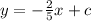
To find c, substitute a coordinate.

when x=6, y= -1,

-1= -⅖(6) +c

`c = -1 + (12)/(5) \\ c = 1 (2)/(5)`

Thus, the equation of the line is

`y = - (2)/(5) x + 1 (2)/(5)`