Answer:
There are 24 ways to select one book of each type.
Explanation:
In mathematics, the procedure to select k items from n distinct items, without replacement, is known as combinations.
The formula to compute the combinations of k items from n is given by the formula:
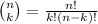
It is provided that there are 6 different biographies and 4 different mystery novels on a bookshelf.
Compute the number of ways to select a biography as follows:
Number of ways to select a biography =
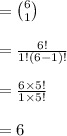
There are 6 ways to select a biography.
Compute the number of ways to select a mystery novel as follows:
Number of ways to select a mystery novel =

There are 4 ways to select a mystery novel.
Then the total number of way to select one book of each type is:

Thus, there are 24 ways to select one book of each type.