Question

The manufacturer of a new compact car claims the miles per gallon (mpg) measurement for the fuel economy is normally distributed with mean =26.7 μ = 26.7 mpg and standard deviation =7.5 σ = 7.5 mpg. You may enter your answers as percents (5% 5 % ) or as real-values (0.05 0.05 ). If you enter your answer as a percent, you must include a % % -sign. Round your answers to the nearest tenth of a percent.

Answer 1

a) 64.26%

b) 4.65%

Explanation:

Data Given :

The mean value μ = 26.7

the standard deviation σ = 7.5

a) The probability that that one new compact car selected at random has a fuel economy of at least 24 mpg is determined as follows:

P(x ≥ 24) = 1- P(x < 24)

P(x ≥ 24) = 1- P( X- μ/σ < 24- 26.7/7.5)

P(x ≥ 24) = 1 - P (Z < -0.36)

P(x ≥ 24) = 1 - 0.3594

P(x ≥ 24) = 0.6426

P(x ≥ 24) = 64.26%

b) Assuming If 30 cars were selected

`P(\bar x > 29) = P((\bar x - \mu )/((\sigma)/(√(n)) ) > (29-26.7)/((7.5)/(√(30) ) ))`

`P(\bar x > 29) = 1-P(Z < 1.68)`

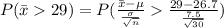
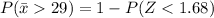
By using Z tables ; we have

`P(\bar x > 29) = 1- 0.9535`

`P(\bar x > 29) =0.0465`

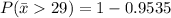
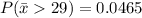
= 4.65%