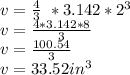Answer:
the volume of the sphere is
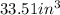
Explanation:
This problem bothers on the mensuration of solid shapes, sphere and cube.
Given data
Volume of cube v = 64 cubic inches
since we are dealing with a cube the height and the radius of the sphere is same as the sides of the cube,
we know that volume of cube is expressed as

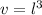
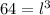
![l= \sqrt[3]{64}](https://img.qammunity.org/2021/formulas/mathematics/high-school/gx9bw61t1cg7o3rozc6nchrfngxxxgvcgp.png)
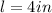
also diameter d=length l
Diameter d=
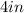
Radius r =
 =
=
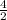 =
=
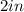
Height h=
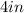
we know that the volume of a sphere is given by
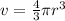
substituting into the formula we have