Answer:
Probability that at most 50 seals were observed during a randomly chosen survey is 0.0516.
Explanation:
We are given that Scientists conducted aerial surveys of a seal sanctuary and recorded the number x of seals they observed during each survey.
The numbers of seals observed were normally distributed with a mean of 73 seals and a standard deviation of 14.1 seals.
Let X = numbers of seals observed
The z score probability distribution for normal distribution is given by;
Z =
 ~ N(0,1)
~ N(0,1)
where,
 = population mean numbers of seals = 73
= population mean numbers of seals = 73
 = standard deviation = 14.1
= standard deviation = 14.1
Now, the probability that at most 50 seals were observed during a randomly chosen survey is given by = P(X
 50 seals)
50 seals)
P(X
 50) = P(
50) = P(


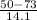 ) = P(Z
) = P(Z
 -1.63) = 1 - P(Z < 1.63)
-1.63) = 1 - P(Z < 1.63)
= 1 - 0.94845 = 0.0516
The above probability is calculated by looking at the value of x = 1.63 in the z table which has an area of 0.94845.