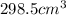Question

A sphere is inscribed in a cube with a surface area of 216 square centimeters. What is the volume of the sphere

Answer 1

`V=298.5cm^3`

Explanation:

To find the volume of the sphere we need to know its radius.

And the radius can be found with the information we have about the surface area.

The formula for the surface area is as follows:

`SA=4\pi r^2`

from this formula we clear the radius r:

`r^2=(SA)/(4\pi)\\ \\r=\sqrt{(SA)/(4\pi) }`

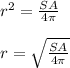
and we substitute the known value of the surface area, and also the value of
`\pi`:

`r=\sqrt{(216cm^2)/(4(3.1416)) }\\ \\r=\sqrt{(216cm^2)/(12.5664) }\\ \\r=√(17.1887cm^2)\\ \\r=4.146cm`

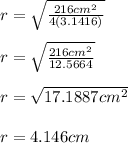
and now that we know the radius we find the volume:

`V=(4\pi r^3)/(3) \\\\V=(4(3.1416)(4.146cm)^3)/(3)\\ \\V=(895.521cm^3)/(3)\\ \\V=298.5cm^3`

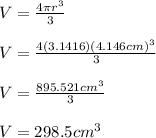
the volume of the sphere is
`298.5cm^3`