Question

Consider the equations and graph below. y = negative 3 (x minus 1). Y = negative 3 x minus 1 On a coordinate plane, 2 lines are parallel to each other. Which explains why this system has no solution? Each has a slope of –3 and a y-intercept of –1, which makes the lines parallel. Each has a slope of –3 and a y-intercept of –1, which makes the lines equivalent. Each has a slope of –3, but one has a y-intercept of 3 and the other has a y-intercept of –1, which makes the lines parallel. Each has a slope of –3, but one has a y-intercept of 3 and the other has a y-intercept of –1, which makes the lines equivalent.

Answer 1

C. Each has a slope of –3, but one has a y-intercept of 3 and the other has a y-intercept of –1, which makes the lines parallel.

Explanation:

For the people who hate reading UuU!

Answer 2

Correct option:

Each has a slope of –3, but one has a y-intercept of 3 and the other has a y-intercept of –1, which makes the lines parallel.

Explanation:

The two equations of straight line are:

`y=-3(x-1)=3x+3\\\\y=-3x-1`

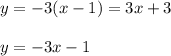
Consider two equations that have the similar slope but different y-intercepts, then the two lines are parallel.

Now since the two equations of parallel lines never intersect each other, the system of these two equations has no solutions.

Thus, the correct reason is:

Each has a slope of –3, but one has a y-intercept of 3 and the other has a y-intercept of –1, which makes the lines parallel.