Answer:
Please refer to attached image for the graph of required circle.
Explanation:
The center and radius equation of a circle is :
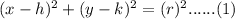
Where the co-ordinates
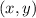 from where the circle passes.
from where the circle passes.
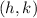 is the co-ordinate of circle's center represented by O.
is the co-ordinate of circle's center represented by O.
 is the radius of circle.
is the radius of circle.
Here, we are given that center of circle is at
 .
.
So,
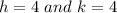 .
.
and
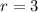
Hence, the equation (1) can be represented as :

Please refer to the image attached in the answer area for the graph of above equation of circle.
Center of circle is represented by O which is the co-ordinate (4,4).
(7,4) is another point on circle.
The radius of circle is also shown in the graph image attached.