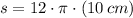Question

De una lamina de 40cm por 60cm se han recortado 6 discos metalicos iguales ,como los de la figura ¿calcula la cantidad de lamina que sobro de recortar los discos si los discos se forran alrededor con un hule de proteccion¿cuantos metros son necesarios

Answer 1

`A_(r) \approx 515.044\,cm^(2)`,
`s\approx 376.991\,cm`

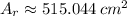Step-by-step explanation:

El área total de la lamina es
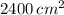`2400\,cm^(2)` (The total area of the sheet is
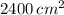`2400\,cm^(2)`). La función asociada al área sobrante es (The function associated with the remaining area is):

`A_(r) = 2400\,cm^(2) - 6\cdot \pi \cdot r^(2)`

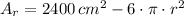
El radio máximo está asociado con la longitud más corta de la lámina. Asúmase que
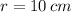`r = 10\,cm` (The maximum radius is associated with the smallest length of the sheet. Let assume that
`r = 10\,cm`):

`A_(r) = 2400\,cm^(2) - 6\cdot \pi \cdot (10\,cm)^(2)`

`A_(r) \approx 515.044\,cm^(2)`

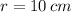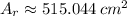
La longitud necesaria de hule de protección es igual a: (The required length of proctection rubber is equal to)

`s = 12\cdot \pi \cdot (10\,cm)`

`s\approx 376.991\,cm`