Question

Solve the following inequality fort:

Answer 1

`$t<1$`

it seems that the answer didn't match the alternatives. But t < 1 is true.

If t > 1:

So, considering t = 2

`$5-4(2)>(3-2)/(2) $`

`$5-8>(1)/(2) $`

`$-3>(1)/(2) $`

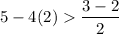
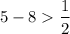
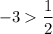
This is not true.

Explanation:

Given inequality:

`$5-4t>(3-t)/(2) $`

`$2(5-4t)>(3-t)/(2) \cdot 2$`

`$10-8t>3-t$`

`$-8t+t>3-10$`

`$-7t>-7$`

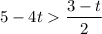
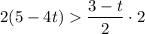
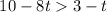
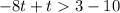
Dividing both sides by -7 will led to the flip of the inequality symbol.

`$t<1$`

Considering t = -1

`$5-4(-1)>(3-(-1))/(2) $`

`$5+4>(3+1)/(2) $`

`$9>(4)/(2) $`

`$9>2 $`

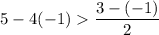
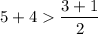
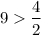
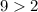
That's right