Question

What is the solution to 2logg(x) = logg8+log,(x-2)?
x=-4
x=-2
X=4
X= 8

Answer 1

The value of x is 4.

Explanation:

You have to use Logarithm Laws :

`log( {a}^(n) ) \: ⇒ \: n log(a)`

`log(a) + log(b) \: ⇒ \: log(a * b)`

So for this question :

`2 log(x) \: ⇒ \: log( {x}^(2) )`

`log(8) + log(x - 2) \: ⇒ \: log(8(x - 2))`

`log( {x}^(2) ) = log(8x - 16)`

Next you have to cut out the log :

`{x}^(2) = 8x - 16`

Then, you have to make the equation equals 0 :

`{x}^(2) - 8x + 16 = 0`

Lastly, you have to solve it :

`{x}^(2) - 4x - 4x + 16 = 0`

`x(x - 4) - 4(x - 4) = 0`

`(x - 4)(x - 4) = 0`

`x = 4`

Answer 2

x = 4

which agrees with the third listed answer among the given options

Explanation:

We can re-write the logarithmic equation using the properties of logarithms:

`2\,log(x)=log(8)+log(x-2)\\log(x^2)=log(8*(x-2))\\log(x^2)=log(8x-16)`

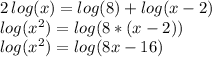
Therefore, the arguments of the log functions must also be equal (and we can solve for "x" by noticing that this expression is the perfect square of a binomial:

`x^2=8x-16\\x^2-8x+16=0\\(x-4)^2=0`

and for this equation to verify, x must be 4