Question

What are the answers & how to solve

Answer 1

Answer:
`√(5)`

Step-by-step explanation:

To solve this, first check to see if the radicands are simplified.

Radicands are the expressions inside the radical symbol.

In this case, we have "20", "45", and "5".

To simplify a radicand, reduce the number to its factors:

20 = 2 * 2 * 5

45 = 3 * 3 * 5

5 = 5

Any factor that appears twice can be simplified by removing it from the radicand and placing it on the left of the radical. For example:

`√(90) = √(3 * 3 * 5 * 2) = 3√(5 * 2)`

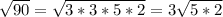
The simplified variant of the square root of 90 is three times the square root of 10.

So, using this method, the simplified versions of the square roots of 20 and 45 are:

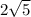`2√(5)` and
`3√(5)`.

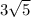Substituting these in the equation:

`-2(2√(5)) + 2(3√(5)) -√(5)`

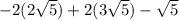
You can multiply the coefficients of the radicals like normal integers -

`-4√(5) + 6√(5) -√(5)`

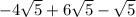
You can treat the square root of 5 as a variable and subtract the coefficients.

-4x + 6x - x = x

The answer is the square root of 5.