Question

Moe hired two separate trios of musicians to play at her tavern on Friday night. Each trio has one piano player, one guitar player, and one sax player. The guitar players have a 87% chance of showing up, the piano players have a 77% chance of showing up, and the saxophone players have a 45% chance of showing up. The two trios know all the same songs so individual musicians can substitute for each other. What is the probability that at least one trio will play on Friday night?

Answer 1

0.6494 or 64.94%

Explanation:

In order for at least one trio to play on Friday night, at least one piano player (P), at least one guitar player (G) and at least one sax player (S) must show up.

The probabilities that at least one of each show up are:

`P(P\geq 1) = 1-P(P=0)\\P(P\geq 1) = 1-(100-77)/(100)*(100-77)/(100) \\P(P\geq 1) =0.9471\\\\P(G\geq 1) = 1-P(G=0)\\P(G\geq 1) = 1-(100-87)/(100)*(100-87)/(100) \\P(G\geq 1) =0.9831\\\\P(S\geq 1) = 1-P(S=0)\\P(S\geq 1) = 1-(100-45)/(100)*(100-45)/(100) \\P(S\geq 1) =0.6975`

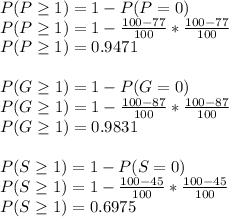
Therefore, the probability that at least one trio will play is:

`P=P(P\geq 1)*P(G\geq 1)*P(S\geq 1) \\P=0.9471*0.9831*0.6975\\P=0.6494`

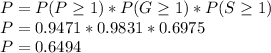
The probability is 0.6494 or 64.94%.