Answer:
81.25% probability that a student is on a sports team given that the student plays a musical Instrument
Explanation:
We use the conditional probability formula to solve this question. It is
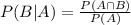
In which
P(B|A) is the probability of event B happening, given that A happened.
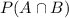 is the probability of both A and B happening.
is the probability of both A and B happening.
P(A) is the probability of A happening.
In this question:
Event A: Playing a musical instrument.
Event B: Being on a sports team.
48% of students play a musical Instrument
This means that
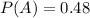
39% of students play a musical Instrument and are on a sports team.
This means that

What is the probability that a student is on a sports team given that the student plays a musical Instrument?
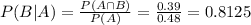
81.25% probability that a student is on a sports team given that the student plays a musical Instrument