Question

From his home, Anthony would have to walk due north to get to his friend Cole's house and

due east to get to his friend Betty's house. It is 6 kilometers from Anthony's house to Betty's

house and a straight-line distance of 10 kilometers from Cole's house to Betty's house. How

far is Anthony's house from Cole's house?

Answer 1

Anthony's house is 8 kilometers from Cole's house

Explanation:

This question can be represented by a rectangle theorem, and we use Pythagoras' Theorem to solve.

We have one vertex for each house.

C

B A

C is Cole's, A is Anthony's and B is Betty's.

6 kilometers from Anthony's house to Betty's

This means that the length of the BA segment is 6 km.

Straight-line distance of 10 kilometers from Cole's house to Betty's house.

This means that the length of the CB segment is 10 k.

How far is Anthony's house from Cole's house?

We need to find CA, which i am going to say that it is x, using the Pythagoras theorem.

So

`x^(2) + 6^(2) = 10^(2)`

`x^(2) = 100 - 36`

`x^(2) = 64`

`x = \pm√(64)`

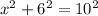
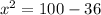
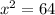
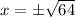
Since it is a distance, we only use the positive value.

`x = 8`

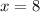
Anthony's house is 8 kilometers from Cole's house