Answer:
We take v in the vector space V different from 0. Since v is not 0, then
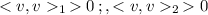
Lets take k>0 such that

Now, we take any vector w. We want to show that
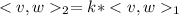 .
.
Since v is any non-zero vector, then this will prove that
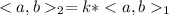 for any vectors a,b. The reason is that for any vector different from 0, lets name it x, there will exist a constant
for any vectors a,b. The reason is that for any vector different from 0, lets name it x, there will exist a constant
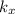 such that
such that
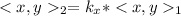 for any y (this is for the same reason a constant exists for v). Since y can be anything, then it can be v. But that means that
for any y (this is for the same reason a constant exists for v). Since y can be anything, then it can be v. But that means that
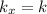 , because v also has its constant k.
, because v also has its constant k.
Now, lets show that
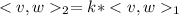 . Lets take a constant c such that
. Lets take a constant c such that
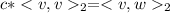 . We have that
. We have that
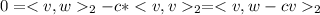
Thus
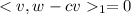
Which means that
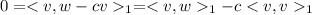
Which means that
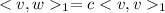 . As a consequence
. As a consequence
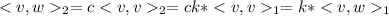
Which proves what we were looking for.