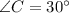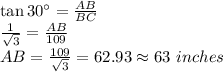Question

When the sun shines at a 30° angle to the ground, Manuel’s shadow is 109 in. long. To the nearest inch, how tall is Manuel?

Answer 1

63 inches

Explanation:

Given: Manuel’s shadow is 109 inches when the sun shines at a 30° angle to the ground.

To find: Height of Manuel

Solution:

Let AB denotes height of Manuel and BC denotes shadow of Manuel.

`\tan C=(side \,\,opposite \,\,to\,\, the\,\, angle)/(side \,\,adjacent \,\,to\,\, the\,\, angle)`

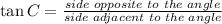
Side opposite to
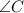`\angle C` = AB

Side adjacent to
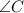`\angle C`= BC

`\angle C=30^(\circ)`

`\tan 30^(\circ)=(AB)/(BC)\\(1)/(√(3))=(AB)/(109)\\AB=(109)/(√(3))=62.93\approx 63\,\,inches`