Answer:28 green and 35 red
Explanation:
Given
If there are r red counter and g green counter then
Probability of drawing a green counter is
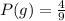
and
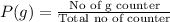
Thus

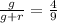
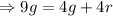
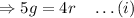
Also if 4 red and 2 green counter is added the probability of drawing a green counter is

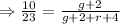
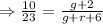

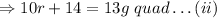
Substitute the value of g in equation (ii)[/tex]
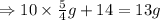
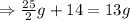
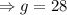
Therefore
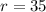
Thus there 28 green counter and 35 red counter