Question

A researcher wishes to​ estimate, with 99​% ​confidence, the population proportion of adults who think the president of their country can control the price of gasoline. Her estimate must be accurate within 3​% of the true proportion. ​a) No preliminary estimate is available. Find the minimum sample size needed. ​b) Find the minimum sample size​ needed, using a prior study that found that 26​% of the respondents said they think their president can control the price of gasoline. ​c) Compare the results from parts​ (a) and​ (b).

Answer 1

a)
`n=(0.5(1-0.5))/(((0.03)/(2.58))^2)=1849`

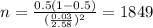
And rounded up we have that n=1849

b)
`n=(0.26(1-0.26))/(((0.03)/(2.58))^2)=1422.99`

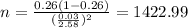
And rounded up we have that n=1423

c) For this case we can see that if we have a prior estimate the minimum sample size required for the margin of error desired would be less as we can see in part b we reduce the sample size compared to the part a

Explanation:

Part a

The critical value for a confidence level of 99% is for this case
`z_(\alpha/2) =2.58`

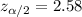
The margin of error for the proportion interval is given by this formula:

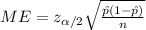`ME=z_(\alpha/2)\sqrt{(\hat p (1-\hat p))/(n)}` (a)

And on this case we have that
`ME =\pm 0.03` and we are interested in order to find the value of n, if we solve n from equation (a) we got:

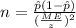`n=(\hat p (1-\hat p))/(((ME)/(z))^2)` (b)

Since we don't know any estimate for the true proportion we can use
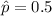`\hat p =0.5` as a godd estimator. And replacing into equation (b) the values from part a we got:

`n=(0.5(1-0.5))/(((0.03)/(2.58))^2)=1849`

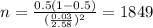
And rounded up we have that n=1849

Part b

For this case we have a prior estimate
`\hat p =0.26` and replacing we got:

`n=(0.26(1-0.26))/(((0.03)/(2.58))^2)=1422.99`

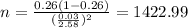
And rounded up we have that n=1423

Part c

For this case we can see that if we have a prior estimate the minimum sample size required for the margin of error desired would be less as we can see in part b we reduce the sample size compared to the part a