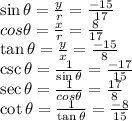Question

Angle (theta) is in standard position. If (8,-15) is on the terminal ray of angle (theta), find the values of the trigonometric functions.

sin(theta)=
cos(theta)=
tan(theta)=
csc(theta)=
sec(theta)=
col(theta)=

What are the answers and how?

Answer 1

`\sin \theta =(-15)/(17)\\cos \theta =(8)/(17)\\\tan \theta =(-15)/(8)\\\csc \theta =(-17)/(15)\\\sec \theta =(17)/(8)\\\cot \theta =(-8)/(15)`

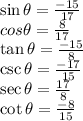
Explanation:

Given: (8,-15) is on the terminal ray of angle

To find: All the trigonometric ratios

Solution:

Trigonometry is a branch of mathematics that explain relationship between the sides and angles of triangles.

If (x, y) lies on the terminal side of θ then
`r=√(x^2+y^2)`

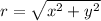
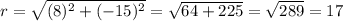`r=√((8)^2+(-15)^2)=√(64+225)=√(289)=17` units

`\sin \theta =(y)/(r)=(-15)/(17)\\cos \theta =(x)/(r)=(8)/(17)\\\tan \theta =(y)/(x)=(-15)/(8)\\\csc \theta =(1)/(\sin \theta )=(-17)/(15)\\\sec \theta =(1)/(cos \theta)=(17)/(8)\\\cot \theta =(1)/(\tan \theta)=(-8)/(15)`