Question

A line, y = mx + b, passes through the point (10, 3) and is parallel to the line 2x+5y=12.

What is the y-intercept

Answer 1

We have been given that a line
`y=mx+b` passes through the point (10, 3) and is parallel to the line
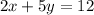`2x+5y=12`. We are asked to find the y-intercept of the line.

First of all we will rewrite our given equation in slope-intercept form as:

`2x-2x+5y=12-2x`

`5y=-2x+12`

`(5y)/(5)=-(2)/(5)x+(12)/(5)`

`y=-(2)/(5)x+(12)/(5)`

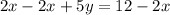
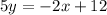
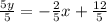
We know that slope of parallel lines is equal, so slope of parallel line to our given line would be
`-(2)/(5)`.

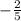Now we will use slope-intercept form of equation to find y-intercept.

`y=mx+b`, where,

m = Slope,

b = The y-intercept.

Let us substitute
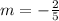`m=-(2)/(5)` and coordinates of point
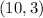`(10,3)` in above equation as:

`3=-(2)/(5)(10)+b`

`3=-2(2)+b`

`3=-4+b`

`3+4=-4+4+b`

`7=b`

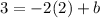
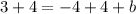
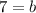
Therefore, the y-intercept is 7 and our required equation would be
`y=-(2)/(5)x+7`.