Answer:
Explanation:
Given
Length of the minute hand = 14 inches
Time = 1 hour
Required
Length of the path traced in 1 hour
For, the minute hand to have completer an hour, this means it completes a cycle;
1 cycle is equivalent to 1 complete rotation;
So, basically the question implies that; the circumference of the complete rotation be solved for;
To solve for this; we make use of the circumference formula;

Where
C represents the circumference
r represents the radius or the length of the minute hand
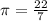 --- This is given from the question;
--- This is given from the question;
By substitution, we have
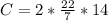
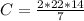
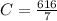
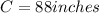
Hence, the length of the path traced is 88 inches