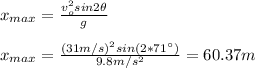Question

A student fires a cannonball diagonally at an angle of 71° with an initial speed of 31m/s. Neglect drag and the initial height of the cannonball.

What was the cannonball's initial horizontal speed?


What was the cannonball's initial vertical speed?


How long did the cannonball rise?


What was the cannonball's total flight time?


What was the cannonball's maximum height?


How far from the cannon did the cannonball land (measured along the ground)?

Answer 1

vx = 10.09 m/s

vy = 29.31 m/s

t = 5.98 s

ymax = 43.83 m

xmax = 60.37 m

Step-by-step explanation:

A) The horizontal speed is constant in the complete trajectory. It is given by:

`v_x=v_ocos\theta\\\\v_x=(31m/s)(cos71\°)=10.09(m)/(s)`

B) The vertical initial speed is:

`v_y=v_osin\theta\\\\v_y=(31m/s)(sin71\°)=29.31(m)/(s)`

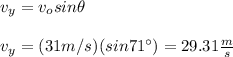
C) The flight time is given by:

`t=(2v_osin\theta)/(g)\\\\t=(2(31m/s)(sin71\°))/(9.8m/s^2)=5.98s`

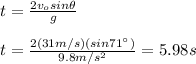
D) The maximum height is:

`y_(max)=(v_o^2sin^2\theta)/(2g)\\\\y_(max)=((31m/s)^2(sin71\°)^2)/(2(9.8m/s))=43.83m`

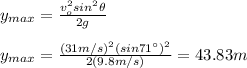
E) The maximum horizontal distance is:

`x_(max)=(v_o^2sin2\theta)/(g)\\\\x_(max)=((31m/s)^2sin(2*71\°))/(9.8m/s^2)=60.37m`