Question

According to the National Institute on Alcohol Abuse and Alcoholism (NIAAA), and the National Institutes of Health (NIH), 41% of college students nationwide engage in "binge drinking" behavior, having five or more drinks on one occasion during the past two weeks. A college president wonders if the proportion of students enrolled at her college that binge drink is lower than the national proportion. In a commissioned study, 462 students are selected randomly from a list of all students enrolled at the college. Of these, 162 admitted to having engaged in binge drinking.

Required:

Using the most conservative estimate, what sample size would be required to reduce the margin of error to 2 percentage points?

Answer 1

The sample size required is 2188.

Explanation:

The (1 - α)% confidence interval for the population proportion is:

`CI=\hat p\pm z_(\alpha/2)\sqrt{(\hat p(1-\hat p))/(n)}`

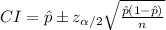
The margin of error for this interval is:

`MOE= z_(\alpha/2)\sqrt{(\hat p(1-\hat p))/(n)}`

The information provided is:

X = 162

n = 462

MOE = 0.02

Assume the confidence level as 95%.

Compute the sample proportion as follows:

`\hat p=(X)/(n)=(162)/(462)=0.351`

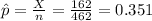
The z-critical value for 95% confidence interval is:

`z_(0.025)=1.96`

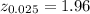
Compute the sample size required as follows:

`MOE= z_(\alpha/2)\sqrt{(\hat p(1-\hat p))/(n)}`

`n=[(z_(\alpha/2)* √(\hat p(1-\hat p)))/(MOE)]^(2)`

`=[(1.96*√(0.351(1-0.351)))/(0.02)]^(2)\\\\=2187.781596\\\\\approx 2188`

Thus, the sample size required is 2188.