Question

f an investor puts $1500 dollars into an account at an annual rate of 4.8% interest compounded twice a month, how much will they have after two years?

Answer 1

They will have $1651 after two years.

Explanation:

The compound interest formula is given by:

`A(t) = P(1 + (r)/(n))^(nt)`

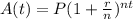
Where A(t) is the amount of money after t years, P is the principal(the initial sum of money), r is the interest rate(as a decimal value), n is the number of times that interest is compounded per unit year and t is the time in years for which the money is invested or borrowed.

$1500 dollars into an account at an annual rate of 4.8%

This means that
`P = 1500, r = 0.048`

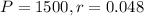
Interest compounded twice a month.

A year has 12 months.

So 12*2 = 24 compundings, which means that
`n = 24`

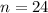
How much will they have after two years?

This is A(2).

`A(t) = P(1 + (r)/(n))^(nt)`

`A(2) = 1500(1 + (0.048)/(24))^(24*2) = 1651`

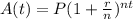
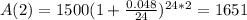
They will have $1651 after two years.