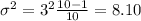Question

In a sample of 10 cabinets, the average height was found to be 37.3in. with a standard deviation of 3.

Give a point estimate for the population variance of the height of the cabinets. Round your answer to two decimal places, if necessary.

Answer 1

`\sigma^2 = 3^2 (10-1)/(10)= 8.10`

Explanation:

For this case we have a sample of n =10 and we have the following statistics:

`\bar X = 37.3 , s= 3`

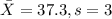
And we want to estimate the population variance. We need to remember that the population variance is given by this formula:

`\sigma^2 =(\sum_(i=1)^n (X_i -\bar X)^2)/(n)`

And the sample variance is given by:

`s^2 =(\sum_(i=1)^n (X_i -\bar X)^2)/(n-1)`

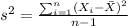
And we can find a formula for the population variance in terms of the sample deviation like this:

`\sigma^2 = s^2 (n-1)/(n)`

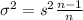
And replacing we got:

`\sigma^2 = 3^2 (10-1)/(10)= 8.10`