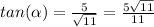Answer:
 and
and
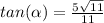
Explanation:
First we would need to solve for the missing leg in order to figure out the remaining answers.
To do this we can use the Pythagorean Theorem a² + b² = c²
Our missing leg in this case is a, so solving for a gives us
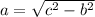
Here our b is 5 and our c is 6. So plugging in these values we get
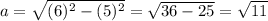
With this missing leg being solved, we can use trig identities to solve for cos and tan
I used the trig identity
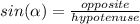 to set up the triangle in the attached image. We can use the trig identity
to set up the triangle in the attached image. We can use the trig identity
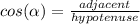 which would give us
which would give us
 and we can use the trig identity
and we can use the trig identity
 which would give us
which would give us