Answer:
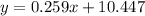
Now we can find the residulls like this:
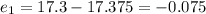
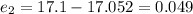
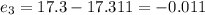


So then we can see that the residuals are not with an specified pattern (alternating sign) so then we can conclude that are distributed normally
Explanation:
We have the following data given:
Height (inches), x 26.75 25.5 26.5 27 25
Head Circumference (inches), y 17.3 17.1 17.3 17.5 16.9
We need to find a linear model
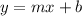
For this case we need to calculate the slope with the following formula:
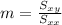
Where:

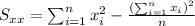
So we can find the sums like this:
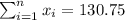
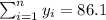
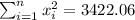
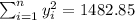
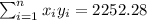
With these we can find the sums:
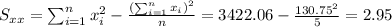
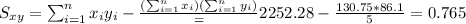
And the slope would be:
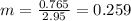
Nowe we can find the means for x and y like this:
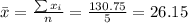
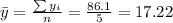
And we can find the intercept using this:
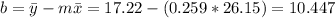
So the line would be given by:
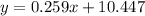
Now we can find the residulls like this:
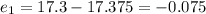
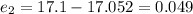
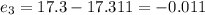


So then we can see that the residuals are not with an specified pattern (alternating sign) so then we can conclude that are distributed normally