Question

The theory of nuclear astrophysics is that all the heavy elements like uranium are formed in the interior of massive stars. These stars eventually explode, releasing these elements into space. If we assume that at the time of the explosion there were equal amount of U-235 and U-238, how long ago did the star(s) explode that released the elements that formed our Earth? The present U-235/U-238 ratio is 0.00700. [The half-lives of U-235 and U-238 are 0.700 × 109 yr and 4.47 × 109 yr.]

Answer 1

t = 5.94x10⁹ years.

Step-by-step explanation:

The time of the explosion can be calculated using the decay equation:

`N_(t) = N_(0)e^(-\lambda t)`

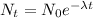
Where:

N(t): is the quantity of the element at the present time

N(0): is the quantity of the element at the time of explosion

λ: is the decay constant

t: is the time

Knowing that the present U-235/U-238 ratio is 0.00700 and that at the time of the explosion there were equal amount of U-235 and U-238, we have:

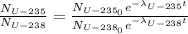`(N_(U-235))/(N_(U-238)) = \frac{N_{U-235_(0)}e^{-\lambda_(U-235) t}}{N_{U-238_(0)}e^{-\lambda_(U-238) t}}` (1)

The decay constant is equal to:

`\lambda = (ln(2))/(t_(1/2))`

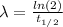
For the U-235 we have:

`\lambda_(U-235) = (ln(2))/(0.700 \cdot 10^(9) y) = 9.90 \cdot 10^(-10) y^(-1)`

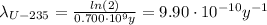
For the U-238 we have:

`\lambda_(U-238) = (ln(2))/(4.47 \cdot 10^(9) y) = 1.55 \cdot 10^(-10) y^(-1)`

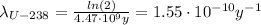
By introducing the values of
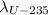`\lambda_(U-235)` and
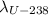`\lambda_(U-238)` into equation (1) we have:

`0.00700 = \frac{e^{-9.90 \cdot 10^(-10) t}}{e^{-1.55 \cdot 10^(-10) t}}`

`0.00700 = e^{(-9.90 \cdot 10^(-10) + 1.55 \cdot 10^(-10)) t}`

`ln(0.00700) = (-9.90 \cdot 10^(-10) + 1.55 \cdot 10^(-10)) t`

`t = (ln(0.00700))/(-9.90 \cdot 10^(-10) + 1.55 \cdot 10^(-10)) = 5.94 \cdot 10^(9) y`

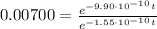
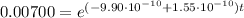
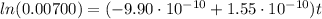
Therefore, the star exploded 5.94x10⁹ years ago.

I hope it helps you!