Question

The​ quality-control manager at a compact fluorescent light bulb​ (CFL) factory needs to determine whether the mean life of a large shipment of CFLs is equal to 7 comma 539 hours. The population standard deviation is 840 hours. A random sample of 49 light bulbs indicates a sample mean life of 7 comma 359 hours. a. At the 0.05 level of​ significance, is there evidence that the mean life is different from 7 comma 539 hours question mark b. Compute the​ p-value and interpret its meaning. c. Construct a 95​% confidence interval estimate of the population mean life of the light bulbs. d. Compare the results of​ (a) and​ (c). What conclusions do you​ reach?

Answer 1

a) Since the p value is higher than the significance level we have enough evidence to FAIL to reject the null hypothesis and we can conclude that the true mean is not significantly different from 7539

b)
`t=(7359-7539)/((840)/(√(49)))=-1.5`

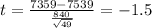
The degrees of freedom are given by:

`df=n-1=49-1=48`

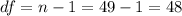
The p value is given by:

`p_v =P(t_((48))<-1.5)=0.07`

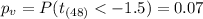
c)
`7359-2.01(840)/(√(49))=7117.8`

`7359+2.01(840)/(√(49))=7600.2`

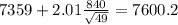
d) For this case since the confidence interval contains the value of 7539 we don't have enough evidence to reject the null hypothesis at the significance level given of 5%. same conclusion using the hypothesis test and with the confidence interval

Explanation:

Part a and b

Data given

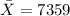`\bar X=7359` represent the sample mean

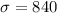`\sigma=840` represent the population standard deviation

`n=49` sample size

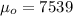`\mu_o =7539` represent the value to test

t would represent the statistic

`p_v` represent the p value

Hypothesis to verify

We want to verify if the mean life is different from 7539 hours, the system of hypothesis would be:

Null hypothesis:
`\mu \geq 7539`

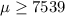
Alternative hypothesis:
`\mu < 7539`

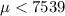
The statistic for this case would be given by:

`t=(\bar X-\mu_o)/((s)/(√(n)))` (1)

Replacing the info given we got:

`t=(7359-7539)/((840)/(√(49)))=-1.5`

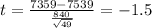
The degrees of freedom are given by:

`df=n-1=49-1=48`

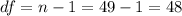
The p value is given by:

`p_v =P(t_((48))<-1.5)=0.07`

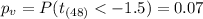
Since the p value is higher than the significance level we have enough evidence to FAIL to reject the null hypothesis and we can conclude that the true mean is not significantly different from 7539

Part c

The confidence interval for the mean is given by the following formula:

`\bar X \pm t_(\alpha/2)(s)/(√(n))` (1)

We can find the critical value using the confidence level given of 95% and using the t distribution with 48 degrees of freedom we got
`t_(\alpha/2)=\pm 2.01`

Now we have everything in order to replace into formula (1):

`7359-2.01(840)/(√(49))=7117.8`

`7359+2.01(840)/(√(49))=7600.2`

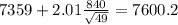
Part d

For this case since the confidence interval contains the value of 7539 we don't have enough evidence to reject the null hypothesis at the significance level given of 5%. same conclusion using the hypothesis test and with the confidence interval