Answer:
240 in²
Explanation:
The prism has an isosceles triangle with the following data:
- Two lengths = 5 inches
- Other length = 8 inches
- Height = 12 inches
Since the prism consist of 2 triangle bases and three rectangular faces, the surface area (SA) of the prism can be calculated as follows:

Where:
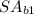 and
and
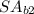 are the surface areas of the two triangle bases.
are the surface areas of the two triangle bases.
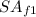 ,
,
 and
and
 : are the surface areas of the three rectangular faces.
: are the surface areas of the three rectangular faces.
The surface area of the triangle bases can be calculated as follows:
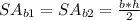
Where:
b: is the base = lenght of 8 inches
h: is the height = 3 inches
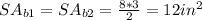
Now, we need to find the surface area of the rectangular faces using the following data:
Rectangular face 1 = rectangular face 2:
- One side = 12 inches
- Other side = 5 inches
Rectangular face 3:
- One side = 12 inches
- Other side = 8 inches
Hence, the SA of the rectangular face 1 and rectangular face 2 is:
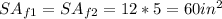
And the SA of the rectangular face 3 is:
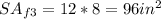
Finally, the SA of the prism is:

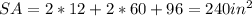
Therefore, the surface area of the prism is 240 in².
I hope it helps you!