Answer:
The dimensions of the vegetable patch is 8 m by 7 m.
Explanation:
Area of a vegetable patch is 56 square meters and the perimeter is 30 m.
If l is length and b is breadth, then area and perimeter of a rectangle is given by :
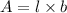

Substituting the values in the above formulas as :
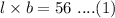
and
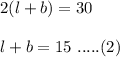
We need to solve equation (1) and (2) to find the values of l and b such that :
Equation (2), l = 15 - b
Equation (1) becomes,
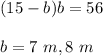
If b = 7 m, so, l = 8 m
If b = 8 m, l = 7 m
So, the dimensions of the vegetable patch is 8 m by 7 m.