Answer:
F = 180 N
Step-by-step explanation:
To find the required force you first calculate the angle of the ramp, by using the following relation:
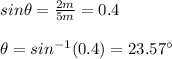
Next, you use the Newton second law to know what is the x component (in a rotated coordinate system) of the gravitational force:
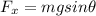
the required force must be, at least, the last force Fx. You know that the weight of the object is 490N = mg. Hence, you have:
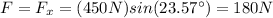
the required force is 180N
- - - - - - - - - - - - - - - - - - - - - - - -
TRANSLATION:
Para encontrar la fuerza requerida, primero calcule el ángulo de la rampa, utilizando la siguiente relación:
Luego, usa la segunda ley de Newton para saber cuál es el componente x (en un sistema de coordenadas girado) de la fuerza gravitacional:
la fuerza requerida debe ser, al menos, la última fuerza Fx. Sabes que el peso del objeto es 490N = mg. Por lo tanto, tienes: